Question

A high school student needs 9 more classes to graduate. If she has met the prerequisites for all the classes, how many possible schedules for next semester could she make if she has 5 class periods to fill? (enter answer with no commas, i.e. as 1000 not 1,000.)

Answer 1

GIVEN

The number of classes needed by the student is 9.

The number of class periods to fill is 5.

SOLUTION

The number of classes she can pick per period is gotten using the combination formula. This multiplied by the total classes will give us the required answer. The answer can thus be calculated as follows:

`N=9C5*5!`

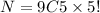
The combination formula is given to be:

`nCr=(n !)/(r ! (n-r) !)`

Therefore, we can calculate the answer to be:

`\begin{gathered} N=(9!)/(5!(9-5)!)*5! \\ N=(9!)/(5!*4!)*5! \\ \text{Simplifying, we have:} \\ N=(9*8*7*6*5*4!*5!)/(5!*4!) \\ N=9*8*7*6*5 \\ N=15120 \end{gathered}`

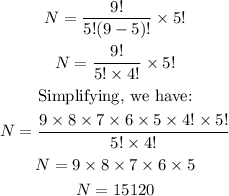
ANSWER

There are 15120 possible schedules that can be made.