Question

Solve the quadratic equation by completing the square. x^2+8x+9=0First choose the appropriate form and fill in the blanks with correct numbers. Then solve the equation. If there is more than one solution, separate with commas.

Answer 1

The quadratic equation given in the question is

`x^2+8x+9=0`

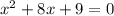
Step 1: Keep x terms on the left and move the constant to the right side by subtracting 9 on both sides

`\begin{gathered} x^2+8x+9=0 \\ x^2+8x+9-9=0-9 \\ x^2+8x=-9 \end{gathered}`

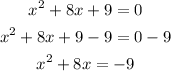
Step 2: Take half of the coefficient of the x term and square it

The coefficient of the x term is 8

`\begin{gathered} (8*(1)/(2))^2 \\ =((8)/(2))^2 \\ =4^2 \\ =16 \end{gathered}`

Step 3: Add 16 to both sides in the equation in step 1

`\begin{gathered} x^2+8x=-9 \\ x^2+8x+16=-9+16 \\ x^2+8x+16=7 \\ \end{gathered}`

Step 4: Re-write the perfect squares on the left

`\begin{gathered} x^2+8x+16=7 \\ (x+4)^2=7 \\ \text{Which will give us } \\ (x+4)^2-7=0 \\ (x+4)^2=7 \end{gathered}`

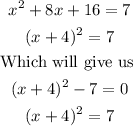
Hence,

We will have (x+4)²=7

To solve for the value of x, we will equate to zeros and make x the subject of the formula

`(x+4)^2=7`

Step 5: Add 7 to both sides

`\begin{gathered} (x+4)^2-7+=0+7 \\ (x+4)^2=7 \end{gathered}`

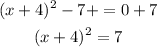
Step 6: Square root both sides

`\begin{gathered} (x+4)^2=7 \\ \sqrt[]{(x+4)}^2=\sqrt[]{7} \\ (x+4)=\pm\sqrt[]{7} \\ \end{gathered}`

Step 7: Isolate the x on the left side and solve for x by subtracting 4 from both sides

`\begin{gathered} (x+4)=\pm\sqrt[]{7} \\ x+4-4=\pm\sqrt[]{7}-4 \\ x=\pm\sqrt[]{7}-4 \\ \text{therefore,} \\ x=-4+\sqrt[]{7} \\ x=-4-\sqrt[]{7} \end{gathered}`

Therefore,

The value of x is

x = -4+√7 , -4-√7