Question

suppose the width of a certain rectangle is 3 inches more than 1/4 of its length. The perimeter of the rectangle is 66 inches. Find the length and width of the rectangle.

Answer 1

Answer:

The length of the rectangle = 24 inches

The width of the rectangle = 9 inches

Explanations:

Let the width of the rectangle be represented by w

Let the length of the rectangle be represented by l

The width is 3 inches more than 1/4 of the length

This can be written mathematically as:

`w\text{ = }(l)/(4)+3\ldots\ldots\ldots\ldots\ldots\text{.}(1)`

The perimeter, p = 66 inches

The perimeter of a rectangle is given by the formula:

p = 2(l + w)

66 = 2(l + w)

l + w = 66/2

l + w = 33

w = 33 - l...........................(2)

Substitute equation (2) into equation (1)

`\begin{gathered} 33\text{ - l = }(l)/(4)+3 \\ \text{Mulitply through by 4} \\ 132\text{ - 4l }=\text{ l + 12} \\ l\text{ + 4l = 132 - 12} \\ 5l\text{ = }120 \\ l\text{ = }(120)/(5) \\ l\text{ = 24} \end{gathered}`

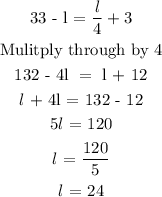
Substitute l = 24 into equation (2)

w = 33 - l

w = 33 - 24

w = 9

The length of the rectangle = 24 inches

The width of the rectangle = 9 inches