Question

Diagram 3 shows a quadrilateral OABC .Point D lies on the line AB.a) Find the length of CDb) A point P moves such that it's distance from point D is always 5 unit. Find the equation of the locus Pc) Find the area of the quadrilateral OABC

Answer 1

a) Length of CD

The length of CD is the distance between point C and point D

`\begin{gathered} C(-7,1)\text{ is the coordinate of point C} \\ D(1,7)\text{ is the coordinate of point D} \end{gathered}`

The distance between two points is calculated by;

`d=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)}`
`\begin{gathered} \text{where;} \\ x_1=-7 \\ y_1=1 \\ x_2=1 \\ y_2=7 \end{gathered}`

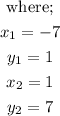
Substituting these values in the formula;

`\begin{gathered} CD=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)} \\ CD=\sqrt[]{(1-(-7))^2+(7-1)^2} \\ CD=\sqrt[]{(1+7)^2+6^2} \\ CD=\sqrt[]{8^2+6^2} \\ CD=\sqrt[]{64+36} \\ CD=\sqrt[]{100} \\ CD=10 \end{gathered}`

Therefore, the length of CD is 10 units

b) The equation of locus P

The locus of points equidistant from a fixed point is a Circle.

Hence, the locus of point P moving at an equal distance of 5 units from point D is a circle with centre D, and radius of 5 units.

The equation of a circle is given by;

`\begin{gathered} (x-h)^2+(y-k)^2=r^2 \\ \text{where;} \\ (h,k)\text{ is the centre of the circle} \\ r\text{ is the radius of the circle} \end{gathered}`

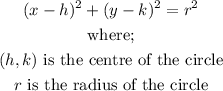
Center D is (1,7)

`\begin{gathered} h=1 \\ k=7 \\ r=5 \end{gathered}`

`\begin{gathered} \text{Substituting into the circle equation,} \\ (x-1)^2+(y-7)^2=5^2 \\ (x-1)^2+(y-7)^2=25 \end{gathered}`

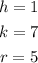
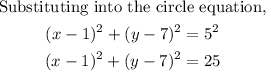
Therefore, the equation of the locus P is;

`(x-1)^2+(y-7)^2=25`

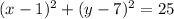
c) Area of quadrilateral OABC.

The area of a polygon can be gotten if the vertices of the polygon are known.

`(\mleft(x_1y_2-y_1x_2\mright) + (x_2y_3-y_2x_3)..... + (x_ny_1-y_nx_1))/(2)`

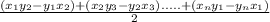
The table below shows the points and the coordinates of the vertices of the polygon.

Applying the formula above by substituting these values into the equation,

`\begin{gathered} ((0-0)+(50-(-25))+(-5-(-70))+(0-0))/(2) \\ =(0+75+65+0)/(2) \\ =(140)/(2) \\ =70 \end{gathered}`

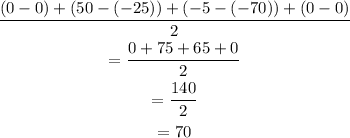
Therefore, the area of the quadrilateral OABC is 70 square units