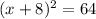Question

What is the intermediate step in the form (x + a)2 = b as a result of completing thesquare for the following equation?6x2 +962 - 13 = -132.Submit Answerattempt 1 out of 2

Answer 1

First step is to separate the terms with variables from the constant terms.

Add 13 to both sides of the equation :

`\begin{gathered} 6x^2+96x-13=-13 \\ 6x^2+96x-\cancel{13}+\cancel{13}=-13+13 \\ 6x^2+96x=0 \end{gathered}`

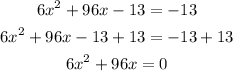
Divide both sides by 6 :

`\begin{gathered} 6x^2+96x=0 \\ x^2+16x=0 \end{gathered}`

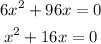
Next step, completing the square by adding this term to both sides of the equation :

`((b)/(2a))^2`

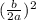
From the equation,

a = 1

b = 16

So it follows that :

`((b)/(2a))^2=((16)/(2*1))^2=(8)^2`

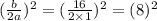
Adding this to both sides of the equation :

`\begin{gathered} x^2+16x=0 \\ x^2+16x+8^2=8^2 \end{gathered}`

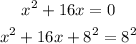
And you will get a perfect square trinomial on the left side of the equation in the form :

`x^2+bx+c^2`

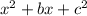
It can be factored as :

`x^2+bx+c^2=(x+c)^2`

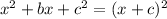
So the equation will be :

`\begin{gathered} x^2+16x+8^2=8^2 \\ (x+8)^2=8^2 \\ (x+8)^2=64 \end{gathered}`

The answer is :

`(x+8)^2=64`