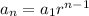Question

Mathematical patterns: Write the first five terms of a sequence. Don’t make your sequence too simple. Write both an explicit formula and a recursive formula for a general term in the sequence. Explain in detail how you found both formulas.

Answer 1

Explanation:

We are to write the first five terms of a sequence, along with the explicit and recursive formula for the general term of the sequence.

Let the first five terms of a sequence be 5, 10, 20, 40 and 80

These terms are taken from a geometric sequence with first term

`a_15,r=2`

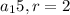
first term a = 5

common ratio r = 2

Therefore, we have

`\begin{gathered} a_2=a_1* r \\ a_3=a_2* r \end{gathered}`

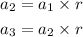
Therefore, the recursive formula is

`\begin{gathered} a_(n+1)=2a_n \\ a_1=5 \end{gathered}`

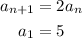
And explicit formula is

`a_n=a_1r^(n-1)`