The function we have is:
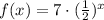
And we need to find which type of function this is.
First, let's find if this is an exponential function or a linear function.
Linear functions have the x-variable multiplied by a coefficient, and exponential functions have the x-variable as an exponent.
In this case, the function is exponential because the variable x is an exponent in the expression.
To choose between options A and C, we need to define if the function is an exponential decay or an exponential growth. To find this, we need to look at the number that has x as the exponent, in this case: 1/2. We define:

As a general rule of a number "a" has the exponent x, the function will be an exponential growth if a>1, and there will be an exponential decay is a is between 0 and 1 0
In this case, 1/2 is between 0 and 1, thus, the function is an exponential decay.
Answer: Exponential decay.