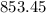Question

Equilateral triangle ABC has a side of length 50.6. Given that M is the intersection of it's medians, determine segment MB times CM to two decimal places.

Answer 1

The product of CM and MB is;

`853.45`

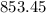
Step-by-step explanation:

Given that the length of each sides of the equilateral triangle is

`50.6`

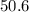
So, the length CB equals 50.6.

Since AD is a bisector then the length CD would be;

`\begin{gathered} CD=(CB)/(2)=(50.6)/(2) \\ CD=25.3 \end{gathered}`

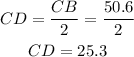
Also, the triangle CDM formed by the bisectors is a right angled triangle.

Since line CE bisect angle ACB.

`\begin{gathered} \measuredangle ACB=60^0\text{ (equilateral triangle)} \\ \measuredangle\text{DCM}=(\measuredangle ACB)/(2)=(60^0)/(2)=30^0 \end{gathered}`

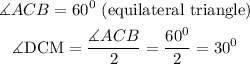
We can then calculate the length of line MB and CM;

`\begin{gathered} \cos (\measuredangle DCM)=(CD)/(CM) \\ \cos 30^0=(25.3)/(CM) \\ CM=(25.3)/(\cos 30^0) \\ CM=29.2139 \end{gathered}`

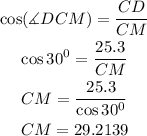
And since it is an equilateral triangle;

`CM=MB=29.2139`

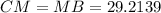
So;

`\begin{gathered} CM* MB=29.2139*29.2139 \\ =853.45 \end{gathered}`

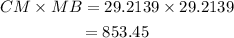
Therefore, the product of CM and MB is;

`853.45`