Question

Use the discriminant to determine the number of x-intercepts of the graph of y = 4x^2-12x + 15. Justify your answer.

Answer 1

Given:

The equation is given as,

`y\text{ = 4x}^2-12x+15`

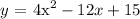
Required:

The number of x intercepts.

Step-by-step explanation:

On comparing the given equation with the standard form of the quadratic equation.

`\begin{gathered} a\text{ = 4} \\ b\text{ = -12} \\ c\text{ = 15} \end{gathered}`

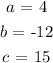
A number of x-intercepts refer to the number of roots of the given quadratic equation.

The discriminant of the given quadratic equation is calculated as,

`\begin{gathered} Discriminant\text{ = b}^2-4ac \\ Discriminant\text{ = \lparen-12\rparen}^2\text{ - 4}*4*15 \\ Discriminant\text{ = 144 - 240} \\ Discriminant\text{ = - 96} \\ \\ As\text{ the discriminant is less than zero therefore the roots of the given } \\ quadratic\text{ equation are imaginary and therefore there are no } \\ no\text{ x - intercepts present.} \\ \\ Answer:\text{ Thus there are no x-intercepts present in the given } \\ quadratic\text{ equation.} \end{gathered}`