ANSWER
The marginal cost for producing 55 golf clubs is $31
Explanation:
Given information
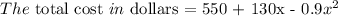
The next step is to find the marginal cost
The formula for finding marginal cost is given below as
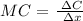
To find the marginal cost, we need to differentiate the total cost with respect to x
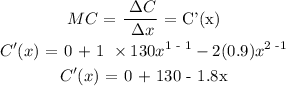
Therefore, the marginal cost is
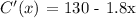
Part b
Find the marginal cost of producing 55 golf clubs
Let x = 55
The next step is to substitute the value of x = 55 into the above marginal cost formula
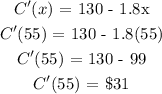
Therefore, the marginal cost for producing 55 golf clubs is $31