Question

At the local burger palace, you can order a hamburger with the following “extras’: tomato, lettuce, bacon, onion, or cheese. How many different ways can you order a burger if you always order one with three different “extras” on it?

Answer 1

10 ways

Step-by-step explanation:

The number of ways to select x elements from a group of n elements is calculated as

`\text{nCx}=(n!)/(x!(n-x)!)`

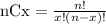
These ways are called combinations. In this case, we need to select 3 different extras from a group of 5 extras ( tomato, lettuce, bacon, onion, or cheese). So, the number of ways to make an order is

`5C3=(5!)/(3!(5-3)!)=(5!)/(3!\cdot2!)=10`

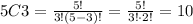
Therefore, the are 10 different ways to order a burger with three different extras.