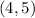Question

What is the solution to the system of equation graphed below?*write your answer as an ordered pair*

Answer 1

Solve by graph

the solution of two lines is the point where they bisect

then , solution point is

`(4,5)`

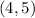
Solve by functions

we write the equation of each line, general equation of a line is

`y=mx+b`

where m is the slope and b the y-intercept

we find the slope using the formula and two points

`m=(y2-y1)/(x2-x1)`

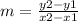
where (x2,y2) is a right point from (x1,y1)

green line

find the slope with points (0,1) and (1,2)

`\begin{gathered} m=(2-1)/(1-0) \\ \\ m=1 \end{gathered}`

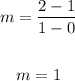
and y-intercept is 1, then b=1

the equation of the green line is

`y=x+1`

yellow line

find the slope with points (-2,2) and (0,3)

`\begin{gathered} m=(3-2)/(0-(-2)) \\ \\ m=(1)/(2) \end{gathered}`

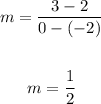
and y-intercept is 3 then b=3

the equation of the yellow line is

`y=(1)/(2)x+3`

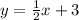
now we have two equations

`\begin{gathered} y=x+1 \\ y=(1)/(2)x+3 \end{gathered}`

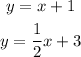
we replace the value of y of any equation on the other equation

i will repalce y from the first equation on the second

`x+1=(1)/(2)x+3`

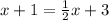
and solve for x

`\begin{gathered} x-(1)/(2)x=3-1 \\ \\ (1-(1)/(2))x=2 \\ \\ (1)/(2)x=2 \\ \\ x=2*2=4 \end{gathered}`

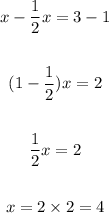
the value of x is 4, now i can repalce x=4 on any equation to find y

i will replace x on the first equation

`\begin{gathered} y=4+1 \\ y=5 \end{gathered}`

the value of y is 5

the point solution is

`(4,5)`