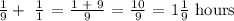Question

Sam and Liam raced each other up and then down the hill. Sam's average speed up the hill was 1 mph, and his average speed down the hill was 9 mph. Lian ran up the hill and down the hill with the same speed, 2 mph. if the path from the bottom to the top of the hill is 1 mile long, how much time did it take each of the boys to finish?

Answer 1

`\begin{gathered} \text{Lian - 1 hour} \\ \text{Sam - 1}(1)/(9)\text{ hours} \end{gathered}`

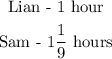
Step-by-step explanation:

Here, we want to calculate the time taken for each of the boys to finish the race

From what we have, the total distance traveled is 2 miles (1 mile up , 1 mile down)

The general formula to get time from speed and distance is:

`\text{time = }\frac{dis\tan ce}{\text{speed}}`

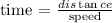
Kindly understand that the journey is in two phases, the leg up and the leg down. The time spent on each will be summed to give the total time spent on the race

Let us start with Lian. We have it as:

`(1)/(2)\text{ + }(1)/(2)\text{ = 1}`

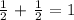
Lian took one hour

For Sam, we have it that:

`(1)/(9)+\text{ }(1)/(1)\text{ = }\frac{1\text{ + 9}}{9}\text{ = }(10)/(9)\text{ = 1}(1)/(9)\text{ hours}`