Question

Molly invests $8,700 into her son‘s college fund, which earns 2% annual interest compounded daily. Find when the value of the fund reaches $12,000.

Answer 1

To answer this question we will use the following formula for compounded daily interest:

`\begin{gathered} A=A_0(1+(r)/(365))^(365t),_{} \\ \text{where A}_0\text{ is the initial amount, r is the annual rate as a decimal number, } \\ \text{and t is the number of years.} \end{gathered}`

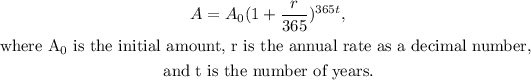
Substituting A₀=8700, A=12000, and r=0.02 we get:

`12000=8700(1+(0.02)/(365))^(365t)\text{.}`

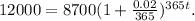
Dividing the above result by 8700 we get:

`\begin{gathered} (12000)/(8700)=(8700)/(8700)(1+(0.02)/(365))^(365t), \\ (40)/(29)=(1+(0.02)/(365))^(365t)\text{.} \end{gathered}`

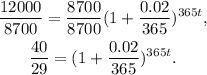
Applying the natural logarithm we get:

`\ln ((40)/(29))=365t\cdot\ln (1+(0.02)/(365))\text{.}`

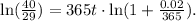
Finally, dividing the above equation by

`365\cdot\ln (1+(0.02)/(365))`

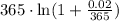
we get:

`t=(\ln((40)/(29)))/(365\ln(1+(0.02)/(365)))\text{.}`

Therefore:

`t\approx16.08`

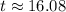
Answer: The value of the fund reaches $12,000 after 16.08 years.