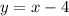Question

Find the equation of each of these lines. (Picture attached)

Answer 1

In order to ginde the line equation for each line, we need to choose two points in each part.

Case 1. Left line (purple)

In this case, we can choose the points

`\begin{gathered} (x_1,y_1)=(0,0) \\ (x_2,y_2)=(1,1) \end{gathered}`

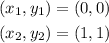
then the slope is given by

`m=(y_2-y_1)/(x_2-x_1)=(1-0)/(1-0)=1`

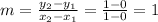
So, we have the following equation

`y=mx+b\Rightarrow y=1\cdot x+b`

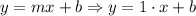
where b is the y-intercept. We can find b by substituting in the last result one of the 2 given points. For instance, if we substitute point (0,0), we have

`\begin{gathered} 0=1\cdot(0)+b \\ 0=0+b \\ \text{then} \\ b=0 \end{gathered}`

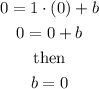
Therefore, the line equation for the purple line is:

`y=x`

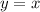
Case 2. Center line (red)

Similarly to the previous case, we need to choose two points along the line, for instance

`\begin{gathered} (x_1,y_1)=(1,1) \\ (x_2,y_2)=(0,2) \end{gathered}`

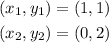
then, by applying the slope formula again, we have

`m=(y_2-y_1)/(x_2-x_1)=(2-1)/(0-1)=(1)/(-1)=-1`

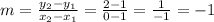
so, the red line has the form

`y=-x+b`

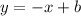
Again, we can find the y-intercept b by substituting one of the 2 given points. If we choose point (0,2), we get

`\begin{gathered} 2=-(0)+b \\ 2=0+b \\ \text{then} \\ b=2 \end{gathered}`

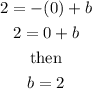
Therefore, the equation of the center line (red) is given by:

`y=-x+2`

Case 3. Blue line

Again, we need to choose 2 points along the line, for instance,

`\begin{gathered} (x_1,y_1)=(3,-1) \\ (x_2,y_2)=(4,0) \end{gathered}`

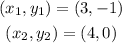
By substituting these point into the slope formula, we have

`m=(y_2-y_1)/(x_2-x_1)=(0-(-1))/(4-3)=(1)/(1)=1`

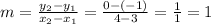
So, the line has the form

`y=1\cdot x+b`

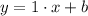
and by substituting the values of the point (4,0) into this equation, we have

`\begin{gathered} 0=1\cdot4+b \\ 0=4+b \\ \text{then} \\ b=-4 \end{gathered}`

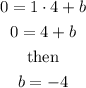
So, the equation of the right line (blue) is given by:

`y=x-4`