Question

Hi! Been out due to medical issues. Trying to learn work so help would be appreciated! Thank you so much.

Answer 1

To answer this question we will set and solve a system of equations.

Let A be the speed (in miles per hour) of the airplane in still air, and W be the wind speed (in miles per hour).

Since the airplane flying with the wind takes 4 hours to travel a distance of 1200 miles and flying against the wind takes 5 hours to travel the same distance, then we can set the following equation:

`\begin{gathered} 4A+4W=1200, \\ 5A-5W=1200. \end{gathered}`

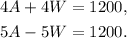
Dividing the first equation by 4 we get:

`\begin{gathered} (4A+4W)/(4)=(1200)/(4), \\ A+W=300. \end{gathered}`

Subtracting A from the above equation we get:

`\begin{gathered} A+W-A=300-A, \\ W=300-A\text{.} \end{gathered}`

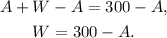
Substituting the above equation in the second one we get:

`5A-5(300-A)=1200.`

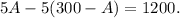
Simplifying the above result we get:

`\begin{gathered} 5A-1500+5A=1200, \\ 10A-1500=1200. \end{gathered}`

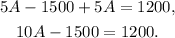
Adding 1500 to the above equation we get:

`\begin{gathered} 10A-1500+1500=1200+1500, \\ 10A=2700. \end{gathered}`

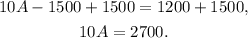
Dividing the above equation by 10 we get:

`\begin{gathered} (10A)/(10)=(2700)/(10), \\ A=270. \end{gathered}`

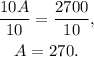
Finally, substituting A=270 at W=300-A we get:

`\begin{gathered} W=300-270, \\ W=30. \end{gathered}`

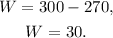
Answer:

The speed of the airplane in still air is 270 miles per hour.

The wind speed is 30 miles per hour.