Question

Find the solution to the following system with algebra. Check by graphing both equations to verify that the lines intersect at the solution you found algebraically, y + 2x = -3 3y - x = 12

Answer 1

Given the set of equations:

y + 2x = -3.......................equation 1

3y - x = 12........................equation 2

Let's find the solution to the system of equations by graphing.

The solution of the system of equations will be the point where the lines of both equations meet (point of intersection).

To graph, rewrite both equations to slope-intercept form: y = mx + b

Where m is the slope and b is the y-intercept.

Equation 1:

y + 2x = -3

Subtract 2x from both sides:

y + 2x - 2x = -2x - 3

y = -2x - 3

Equation 2:

3y - x = 12

Add x to both sides:

3y - x + x = x + 12

3y = x + 12

Divide all terms by 3:

`\begin{gathered} (3y)/(3)=(x)/(3)+(12)/(3) \\ \\ y=(1)/(3)x+4 \end{gathered}`

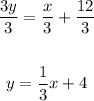
Thus, we have both equations in slope intercept form:

y = -2x - 3

y = ⅓x + 4

We have the graph below:

From the graph of both equations above, we can see the point both lines intersect is at the point: (x, y) ==> (-3, 3)

Where:

x = -3, y = 3

Therefore, the solution to the system of equations is (-3, 3)

ANSWER:

(-3, 3)