Question

The area of a rectangular wall on a barn is 80 ft². Its length is 6 feet longer than twice its width. Find the length and width of the wall of the barn.

Answer 1

The area of the rectangular barn is 80 ft².

Let "l" represent the length of the barn and "w" represent the width.

You know that the length is 6ft longer than twice the width.

The length of the wall can be expressed as follows:

`l=2w+6`

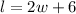
The area of the rectangular wall is equal to the product of the length and width:

`A=lw`

Write the formula using A=80 and l=2w+6

`\begin{gathered} A=lw \\ 80=(2w+6)w \end{gathered}`

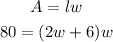
Distribute the multiplication on the parentheses term:

`\begin{gathered} 80=(2w+6)w \\ 80=2w*w+6*w \\ 80=2w^2+6w \end{gathered}`

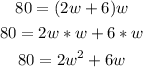
Zero the equation by subtracting 80 to both sides of the equal sign:

`\begin{gathered} 80-80=2w^2+6w-80 \\ 0=2w^2+6w-80 \end{gathered}`

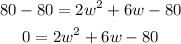
The expression obtained is a quadratic equation, to determine the possible values of "w" you have to use the quadratic formula:

`x=(-b\pm√(b^2-4ac))/(2a)`

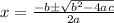
Where

a is the coefficient of the quadratic term

b is the coefficient of the x-term

c is the constant

For this exercise, the independent variable is "w" and the coefficients are:

a=2

b=6

c=-80

`\begin{gathered} x=(-b\pm√(b^2-4ac))/(2a) \\ w=(-6\pm√(6^2-4*2*(-80)))/(2*2) \end{gathered}`

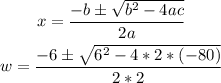
Simplify as much as possible:

`\begin{gathered} w=(-6\pm√(6^2-4*2*(-80)))/(2*2) \\ w=(-6\pm√(36-640))/(4) \\ w=(-6\pm√(676))/(4) \\ w=(-6\pm26)/(4) \end{gathered}`

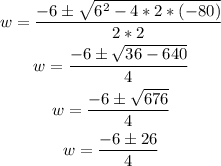
Next, solve the sum and difference separately:

- Sum:

`\begin{gathered} w=(-6+26)/(4) \\ w=(20)/(4) \\ w=5ft \end{gathered}`

-Difference

`\begin{gathered} w=(-6-26)/(4) \\ w=-(32)/(4) \\ w=-8ft \end{gathered}`

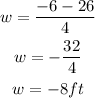
The possible values of w are 5ft and -8ft.

Now, the width is a dimension, and thus it cannot be negative. So, although w=-8ft is mathematically correct, it is not a valid value for the width of the wall.

Then, the width of the wall is w=5ft.