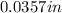The area of a circle with radius r is given by the formula:
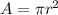
If we change the value of r by a small amount Δr, the new value of the area will be:
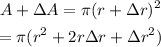
We can neglect the term Δr^2 since we are assuming that Δr is a very small quantity. Then:
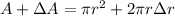
Substitute A=πr^2 and isolate Δr from the equation:
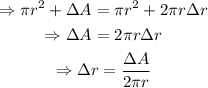
Assuming that the ideal area of the disk is 1000in^2, calculate the ideal radius of the disk:
![\begin{gathered} A=\pi r^2 \\ \Rightarrow r^2=(A)/(\pi) \\ \Rightarrow r=\sqrt[]{(A)/(\pi)} \\ \Rightarrow r=\sqrt[]{(1000in^2)/(\pi)} \\ \Rightarrow r=17.84124116\ldots in \end{gathered}](https://img.qammunity.org/qa-images/2023/formulas/mathematics/college/szjswb7v2hsj29hi1ll8.png)
Substitute the value of r as well as the variation on the value of the area ΔA=4in^2 to find the variation in the value of the radius:
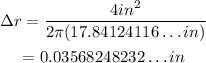
Up to 3 significant figures, the variation in the value of the radius must be less than: