Question

a chef is going to use a mixture of two brands of Italian dressing. the first brand contains 5% vinegar, and the second brand contains 8% vinegar. the chef wants to make 300 milliliters of a dressing that is 6% vinegar. how much of each branch should she use?First brand: __milliliters Second brand: __milliliters

Answer 1

Let A be the first brand and B the second brand, then, we can write

`\begin{gathered} 0.05A+0.08B=0.06*300\ldots(a) \\ \text{and} \\ A+B=300\ldots(b) \end{gathered}`

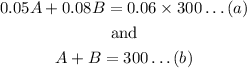
so we have 2 equations in 2 unknows.

Solving by substitution method.

By moving B to the right hand side in the second equation, we have

`A=300-B\ldots(c)`

By substituting this result into equation (a), we have

`0.05(300-B)+0.008B=18`

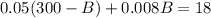
where 18 = 0.06x300. By combining similar terms, we get

`\begin{gathered} 15-0.05B+0.08B=18 \\ 15+0.03B=18 \end{gathered}`

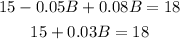
By moving 15 to the right hand side, we obtain

`\begin{gathered} 0.03B=18-15 \\ 0.03B=3 \end{gathered}`

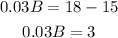
then, B is equal to

`\begin{gathered} B=(3)/(0.03) \\ B=100 \end{gathered}`

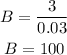
Now, by substituting this result into equation (c), we have

`\begin{gathered} A=300-100 \\ A=200 \end{gathered}`

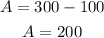
This implies tha the answer is:

First brand: 200 mililiters

Second brand: 100 mililiters