As mentioned in the question, Line a and Line b are parallel to each other and these two lines are cut by the transversal line c.
Since line b is cut by the transversal line c forming the angles measuring 64° and y°, these angles are called linear pairs. The sum of the angles of a linear pair is 180°. Hence, we can say that:

From that equation, we can solve for the value of y.
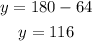
Therefore, the value of y is 116°.
On the other hand, angle x and angle y are what we call alternating exterior angles. By definition, alternating exterior angles are congruent. Hence, the value of x is also 116°.
Why are x and y alternating exterior angles? X and Y are alternating exterior angles because first, they are angles found outside lines a and b, hence, the word exterior. Also, angles x and y do not lie on the same side of the transversal. Hence, the word alternating.