Question

In 5-card poker, find the probability of being dealt the following hand. Refer to thetable. Note that a standard deck of playing cards has 52 cards—4 suits (clubs,diamonds, hearts, spades), where each suit has 13 cards (Ace, 2,3,4,5,6,7,8,9. 10. Jack Queen King).four fives

Answer 1

0.00001847

Explanation:

A hand consists of 5 cards from a well shuffled deck of 5 cards.

Which means that there is a total number of outcomes = 52C5

`\begin{gathered} _(52)C_5=(52!)/(5!\cdot47!) \\ \\ _(52)C_5=2598960 \end{gathered}`

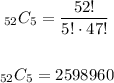
Since we get 4 fives, number of favorable outcomes of a it would be 48 (52 - 4)

Therefore, the required probability is:

`\begin{gathered} p=\frac{\text{ number of favorable outcomes}}{\text{ total number of outcomes }} \\ \\ \text{ we replacing:} \\ \\ p=(48)/(2598960)=0.00001846892\cong0.00001847 \end{gathered}`

The probability of being dealt four fives is 0.00001847