Question

suppose that the heights of adult men in th united states are normally distributed with a mean of 70 inches and a standard deviation of 3 inches. What proportion of the adult men in the united states are less than 6 feet tall?

Answer 1

Answer:

Proportion of the adult men less than 6 feet tall = 74.857%

Explanations:

The mean height, μ = 70 inches

The standard deviation in height, σ = 3 inches

Proportion of the adult men less than 6 feet

1 foot = 12 inches

6 feet = 12 x 6 = 72 inches

x = 72 inches

Calculate the z-value using the formula below:

`\begin{gathered} z\text{ = }\frac{\text{x -}\mu\text{ }}{\sigma} \\ z\text{ = }(72-70)/(3) \\ \text{z = }(2)/(3) \\ \text{z = }0.67 \end{gathered}`

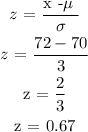
Probability that an adult men will be less than 72 inches (6 feet) tall

P(x < 72) = P(z < 0.67) = 0.74857

Therefore, proportion of adult men less than 72 inches (6 feet) tall = 0.74857 x 100% = 74.857%