Question

Find an equation of variation in which y varies directly as x and y=8 when x=9. Then find the value of y when x=72

Answer 1

The question involves a direct variation between x and y,therefore

`\begin{gathered} y\text{ }\alpha\text{ x} \\ y=kx\ldots\ldots\ldots\ldots\text{.}(1) \end{gathered}`

Given that,

`\begin{gathered} y=8 \\ \text{when } \\ x=9 \end{gathered}`

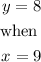
Substitute the values of x and y in equation (1)

`\begin{gathered} y=kx \\ 8=k*9 \\ 8=9k \\ (9k)/(9)=(8)/(9) \\ k=(8)/(9) \end{gathered}`

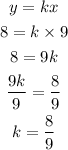
Substituting the value of k=8/9 in equation (1), we will have the equation of variation to be

`\begin{gathered} y=kx,\text{ becomes} \\ y=(8)/(9)x \end{gathered}`

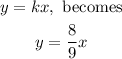
Hence,

The equation of the variation is y= 8x/9

Next, we will calculate the value of y when x=72 using the equation of variation above

`\begin{gathered} \text{equation of variation is} \\ y=(8)/(9)x \\ \text{when x=72,we will have y to be} \\ y=(8)/(9)*72 \\ y=(576)/(9) \\ y=64 \end{gathered}`

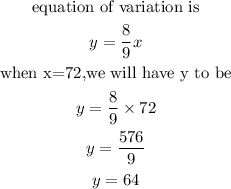
Hence,

The value of y when x=72 is y=64