Question

We need to find the standard form of the equation of each hyperbolas

Answer 1

y^2/ 25 - x^2 / 9 = 1

Explanation:

Th standard form of a hyperbola is

`((y-h)^2)/(a^2)-((x-k)^2)/(b^2)=1`

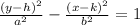
where (h,k ) are the coordinates of the centre and a and b are two constants.

The coordinates of the vertices are

`(h,k\pm a)`

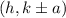
and the equation of the asymptote is

`y-(a)/(b)(x-h)+k`

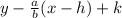
Now, the coordinates of the center for our hyperbola are (0,0); therefore,

`(h,k)=(0,0)`

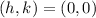
and the coordinates of the vertices we get from the graph are

`(h,k\pm a)=(0,\pm5)`
`\therefore a=5`

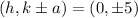
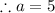
Finally, the equation for the asymptote we get from the graph is

`y=(5)/(3)x`

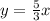
meaning

`b=3`

Hence,

h = 0,

b = 0,

a = 5,

b = 3

thereofore, the equation of the hyperbola is

`((y-0)^2)/(5^2)-((x-0)^2)/(3^2)=1`
`(y^2)/(25)-(x^2)/(9)^{}=1`

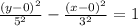
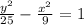
which is our answer!