Question

Help me please, I tried this one but immediately got lost

Answer 1

Given:

The general equation of a circle is,

`7x^2+7y^2-28x+42y-35=0\text{ ------(1)}`

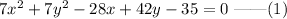
The standard equation of a circle is given by,

`(x-h)^2+(y-k)^2=r^2\text{ ------(2)}`

Here, (h, k) is coordinates of the center of the circle and r is the radius of the circle.

Equation (1) can be converted to the standard form by first dividing equation (1) by 7.

`x^2+y^2-4x+6y-5=0\text{ }`

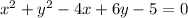
Grouping the x's and y's in the above equation,

`x^2-4x+y^2+6y=5`

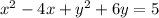
Complete the square of variables x and y by adding constants that create prefect square trinomials.

`\begin{gathered} x^2-4x+4+y^2+6y+9=5+4+9 \\ x^2-2* x*2+2^2+y^2+2* y*3+3^2=18 \end{gathered}`

Now, factor the perfect square trinomials in each variable.

`\begin{gathered} (x-2_{})^2+(y+3)^2=(\sqrt[]{18})^2 \\ (x-2_{})^2+(y+3)^2=(3\sqrt[]{2})^2 \end{gathered}`

So, the equation of the circle in standard form is,

`(x-2_{})^2+(y+3)^2=(3\sqrt[]{2})^2`

Comparing the above equation with the standard form of the equation of a circle given in equation (2), we get

`h=2,\text{ k=-3 and r=3}\sqrt[]{2}`

Therefore, the center of the circle is at the point (2, -3) .

The radius of the circle is,

`3\sqrt[]{2}`