Question

What is the probability of rolling a number less than or equal to 8 with two dice, given that at least one of the dice must show a 6?

Answer 1

If one die shows 6, the second can be a maximum of 8-6=2. This leads to a probability of 2/6=1/3.

Answer 2

`(1)/(9)`

Explanation:

Events :

{1,1} ;{1,2};{1,3};{1,4};{1,5};{1,6}

{2,1} ;{2,2};{2,3};{2,4};{2,5};{2,6}

{3,1} ;{3,2};{3,3};{3,4};{3,5};{3,6}

{4,1} ;{4,2};{4,3};{4,4};{4,5};{4,6}

{5,1} ;{5,2};{5,3};{5,4};{5,5};{5,6}

{6,1} ;{6,2};{6,3};{6,4};{6,5};{6,6}

Total events = 36

Favorable events = Sum of number less than or equal to 8 with two dice, given that at least one of the dice must show a 6 = {1,6};{2,6};{6,1} ;{6,2} =4

Probability of getting an event =
`\frac{\text{Favorable events}}[\text{Total events}}`

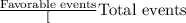
So, probability of rolling a number less than or equal to 8 with two dice, given that at least one of the dice must show a 6 =
`(4)/(36)`

=
`(1)/(9)`

Thus , the probability of rolling a number less than or equal to 8 with two dice, given that at least one of the dice must show a 6 is
`(1)/(9)`