Question

Show tan (x) + cot (x) • sec(x)

Answer 1

`\tan x+\cot x=\csc x.\sec x`

Let us change tan x and cot x to sin x and cos x

`\begin{gathered} \because\tan x=(\sin x)/(\cos x) \\ \because\cot x=(\cos x)/(\sin x) \end{gathered}`

Substitute them on the left side

`LHS=(\sin x)/(\cos x)+(\cos x)/(\sin x)=(\sin^2x+cox^2x)/(\cos x\sin x)`

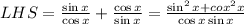
I multiplied the denominators and multiply each numerator by the opposite denominator

`\because\sin ^2x+cox^2x=1`
`\therefore L.H.S=(1)/(\sin x\cos x)`

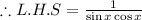
Now we will work on the right hand side

`\begin{gathered} \because\csc x=(1)/(\sin x) \\ \because\sec x=(1)/(\cos x) \end{gathered}`

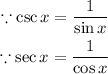
Substitute them on the right side

`\because R.H.S=(1)/(\sin x)*(1)/(\cos x)=(1)/(\sin x\cos x)`

The L.H.S = R.H.S = 1/(sin x cos x)