Question

Can you help me please I need help with this assignment

Answer 1

a) Consider the experiment of picking 3 tax returns at random as 3 related experiments (due to the fact that it is without replacement); then, as for the first time one selects a tax return,

`P_1(NonError)=(61)/(61+9)=(61)/(70)`

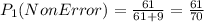
As for the second time we grab a tax return, there are 69 tax returns in total and 9 of them contain errors; thus,

`P_2(NonError)=(60)/(69)`

Similarly, as for the third picking round,

`P_3(NonError)=(59)/(68)`

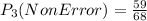
Finally, the probability of experiment a) is

`\begin{gathered} P_1(NonError)*P_2(NonError)*P_3(NonError)=(61*60*59)/(70*69*68)=0.657471...\approx65.7\% \\ \end{gathered}`

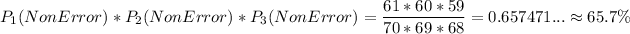
Rounded to one decimal place, the probability of event a) is 65.7%

b) Similarly, in the event of all three tax returns containing errors,

`\begin{gathered} P_1(Error)=P_1(E)=(9)/(70) \\ P_2(E)=(8)/(69) \\ P_3(E)=(7)/(68) \end{gathered}`

Thus,

`\begin{gathered} \Rightarrow P(b)=P_1(E)*P_2(E)*P_3(E)=0.00153... \\ \Rightarrow P(b)\approx0.2\% \end{gathered}`

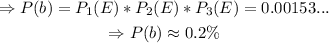
The probability of event b) is 0.2%. It is quite unusual.

c) The condition 'at least one of those containing errors' includes the cases when 1, 2, or 3 tax returns of the ones selected have errors. Notice that

`1=P(0Errors)+P(1Errors)+P(2Errors)+P(3Errors)`

Therefore,

`P(1o2o3Errors)=P(1E)+P(2E)+P(3E)=1-P(0Errors)`

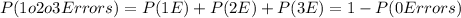
And we found the probability of picking 3 tax returns without any errors in part a); thus,

`P(c)=1-0.657471...=0.342528...\approx34.3\%`

The probability of event c) is 34.3%.

d) Analogously to part c), the probability of selecting at least one without errors is

`P(d)=1-P(0NonErrors)=1-P(3Errors)=1-P(b)=0.998465...\approx99.8\%`

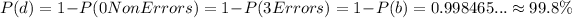
The probability of event d) is 99.8%, and it is not improbable at all.