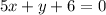Question

Find the equation of the line that passes through the two points ( -3,9) and ( -1/2,-7/2).Write your answer in standard form.

Answer 1

Given

The line passes through the points,

`(-3,9),(-(1)/(2),-(7)/(2))`

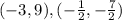
To find the equation of the line and write in the standard form.

Now,

The equation of the line passing through two points is given by,

`\frac{y_{}-y_1}{y_2-y_1}=\frac{x_{}-x_1}{x_2-x_1}`

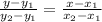
Since the points are

`(-3,9),(-(1)/(2),-(7)/(2))`

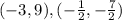
Then, the equation of the line is,

`\begin{gathered} (y-9)/(-(7)/(2)-9)=(x-(-3))/(-(1)/(2)-(-3)) \\ (y-9)/((-7-18)/(2))=(x+3)/((-1+6)/(2)) \\ (y-9)/(-25)=(x+3)/(5) \\ (y-9)/(-5)=x+3 \\ y-9=-5(x+3) \\ y-9=-5x-15 \\ y=-5x-15+9 \\ y=-5x-6 \end{gathered}`

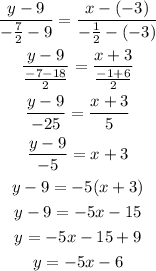
Hence, the equation of the line is,

`y=-5x-6`

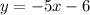
And, the standard form of the line is,

`5x+y+6=0`