Question

What are the steps to solving the inequality 3b + 8 ≥ 14

Answer 1

3b+8≥14
Subtract 8 from both sides
3b≥14-8
3b≥6
Divide both sides by 3
b≥6/3
b≥2

Answer 2

The solution of the given inequality
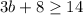`3b+8\geq 14` is:
`[2, \infty)`

Explanation:

Given the inequality equation:
`3b+8\geq 14`

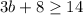
Subtraction property of equality states that you subtract the same number to both sides of an equation.

Subtract 8 to both sides of an equation.

`3b+8-8\geq 14-8`

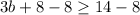
Simplify:

`3b\geq 6`

Division property of equality states that you divide the same number to both sides of an equation.

Divide both sides by 3 we get;

`(3b)/(3) \geq (6)/(3)`

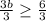
Simplify:

`b\geq 2`

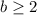
therefore, the solution of the given inequality is:
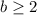`b\geq 2` or
`[2, \infty)`