28.06 metres
Step-by-step explanation
Step 1
find the elastic potential energy
it is given by:
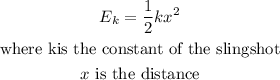
so
convert the measure into meteres and kg

so
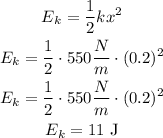
Step 2
now, use the conservation of energy law to find the maximum heigth
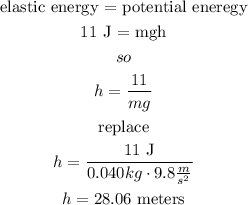
therefore, the answer is
28.06 metres
I hope this helps you