We need to find the surface area of a cylinder with a radius of 8 yd and a height of 6 yd. This cylinder and its lateral surface are shown below:
Notice that its lateral surface corresponds to a rectangle with a width equal to the height of the cylinder, and a length equal to the perimeter of the cylinder's base.
Thus, the lateral surface area is given by:
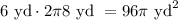
And the area of each base is the area of a circle with a radius of 8 yd:
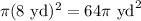
Since it has a top and a bottom base, we need to multiply the last result by two and then add it to the lateral surface area to find the total surface area. We obtain:
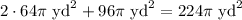
If we approximate the answer to one decimal place, we obtain:

Answer: Approximately 703.7 yd²