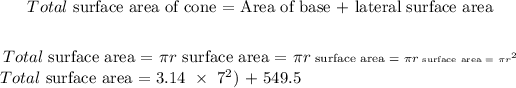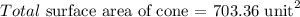Answer:

Step-by-step explanation:
Given:
AB = 14
SO = 24
To find:
a) the volume
b) the area of the lateral surface
c) the total surface area
a) To find the volume of the cone, we will use the formula:
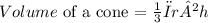
r = radius
diameter = AB = 14
diameter = 2(radius)
radius = diameter/2 = 14/2
radius = 7
height = SO = 24
let π= 3.14

b) To get the lateral surface, we will apply the formula:
![\begin{gathered} lateral\text{ surface of the cone = \pi rl} \\ where\text{ l = }√(h^2+r^2) \end{gathered}]()
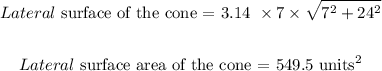
c) The total surface area formula: