Given:
The distance traveled by helicopter against the headwind, d₁=450 miles
The speed of the wind, w=35 mph
The distance traveled by helicopter the tailwind, d₂=702 miles
To find:
The speed of the helicopter.
Step-by-step explanation:
Let us assume that the time it takes for the helicopter to travel the distances is t
The time duration for an object to cover a distance is given by,
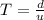
Where t is the time, d is the distance, and u is the velocity.
As the time it takes for the helicopter to cover the distances d₁ and d₂ are the same,

Where v-w is the total speed of the helicopter when it is flying against the headwind and v+w is the speed of the helicopter when it is flying with a tailwind.
On substituting the known values,

Final answer:
Thus the speed of the helicopter is 160 m/s