Question

Determine the roots algebraically by factoring:4x^4+ 6x^3- 6x^2 -4x=0

Answer 1

we have the equation

`4x^4+6x^3-6x^2-4x=0`
`x(4x^3+6x^2-6x^{}-4)=0`
`(4x^3+6x^2-6x^{}-4)=0`

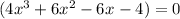
Note that

For x=1

`\begin{gathered} (4(1)^3+6(1)^2-6(1)^{}-4)=0 \\ 0=0 \end{gathered}`

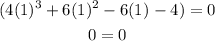
x=1 is a root of the given equation

so

Divide

(4x^3+6x^2-6x-4) : (x-1)

4x^2+10x+4

-4x^3+4x^2

---------------------------

10x^2-6x-4

-10x^2+10x

-----------------------

4x-4

-4x+4

-----------

0

therefore

(4x^3+6x^2-6x-4)=(x-1)(4x^2+10x+4)

Solve the quadratic equation

(4x^2+10x+4)=0

a=4

b=10

c=4

substitute in the formula

`x=\frac{-10\pm\sqrt[]{10^2-4(4)(4)}}{2(4)}`
`x=\frac{-10\pm\sqrt[]{36}}{8}`

the values of x are

x=-1/2 and x=-2

therefore

(4x^2+10x+4)=4(x+1/2)(x+2)=(4x+2)(x+2)

and the answer is

x(x-1)(4x+2)(x+2)=0