Answer:
4.73 m/s²
Step-by-step explanation:
The free body diagram for the bucket is
Then, the net force is equal to
Fnet = T - mg = ma
Where T is the tension of the rope, m is the mass, g is the gravity, and a is the acceleration. Solving for a, we get:
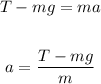
Replacing T = 20.2 N, m = 1.39 kg, and g = 9.8 m/s², we get

Therefore, the acceleration is 4.73 m/s²