Question

The diagonal of a square is 2 inches.Find the area of the square.

Answer 1

in a 45 45 90 triangle the sides are x by x by x*sqrt(2) respectively

therefore x*sqrt(2) = 2 [the 2 that was mentioned in the question]

solving for x
x = 2 / sqrt(2) = sqrt(2) [after rationalizing the denominator]

x = sqrt(2) is now our side length

Area of Square = (side length)^2
so the Area of this Square = (sqrt(2))^2 = 2

therefore your answer is 2

Answer 2

Since this square has a diagonal length of 2 inches, the area of the square is equal to 2 square inches.

In Mathematics and Geometry, the area of a square can be calculated by using this mathematical equation (formula);

A =
`x^2`

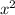
Where:

• A represents the area of a square.
• x represents the side length of a square.

In Mathematics and Geometry, the side length of a square can be calculated by using this mathematical equation (formula);

Diagonal, d = √2x

By solving for x, we have the following side length:

x = d/√2

x = 2/√2

x = meters.

Now, we can determine the area of this square;

Area of square =
`((2)/(√(2) ) )^2`

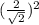
Area of square = 4/2

Area of square = 2 square inches.