Question

POSSI 20 2 13 In the above triangle, would you use SINE, COSINE, or TANGENT to solve for the missing side (TYPE IN ALL CAPS)? The measure of the missing angle is (ROUND TO THE NEAREST DEGREE). 1 2 3 4 5 6

Answer 1

Given:

The measure of the hypotenuse of the right triangle is 20.

The measure of another side of the right triangle is 13.

The objective is to find the missing angle.

Step-by-step explanation:

Consider the missing angle as θ. With respect to the missing angle, the measure of side 13 is the adjacent side.

Considering the adjacent and hypotenuse the missing angle can be calculated by COSINE.

To find θ:

The measure of missing angle can be calculated as,

`\text{COSINE}\theta=\frac{\text{adjacent}}{\text{hypotenuse}}\text{ . . . . . .(1)}`

On plugging the given values in equation (1),

`\text{COSINE}\theta=(13)/(20)`

On further solving the above equation,

`\begin{gathered} \theta=COSINE^(-1)((13)/(20)) \\ \theta=49.4583981\ldots.. \\ \theta\approx49\degree \end{gathered}`

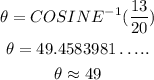
Hence, the measure of the missing angle is 49°.