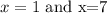Question

What is the quotient (4-x)/(x^2+5x-6) divided (x^2 - 11x + 28) / (x^2 + 7x +6) in simplified form? State any restrictions on the variable.See image

Answer 1

Step-by-step explanation:

To solve the question, we will have to get the factor of the terms given

Let us follow the steps below

Step 1: Write out the given expression

`(4-x)/(x^2+5x-6)/(x^2-11x+28)/(x^2+7x+6)`

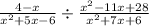
Step 2: simplify each term by writing them in factor form

`(4-x)/((x-1)(x+6))/((x-4)(x-7))/((x+1)(x+6))`

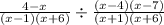
Step 3: Re-write the expression

`(4-x)/((x-1)(x+6))*((x+1)(x+6))/((x-4)(x-7))`

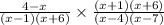
Step 4: Simplify further and cancel out like terms

`(4-x)/(x-1)*(x+1)/((x-4)(x-7))`

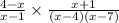
Step 5: simplify by factoring out the negative sign

`(-(x-4))/(x-1)*(x+1)/((x-4)(x-7))`

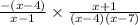
Step 6: Resolve the expression

`(-1(x+1))/((x-1)(x-7))`

Thus, we have the simplified form to be

`(-x-1)/((x-1)(x-7))`

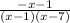
Then for the restrictions, these will be values of x which makes it undefined

These values are

`x=1\text{ and x=7}`