The length of the professor's classes is uniformly distributed with a minimum length of a=50.0min and a maximum length of b=52.0min.
To determine the asked probability you have to work using the cumulative distribution function which can be defined for this distribution as follows:
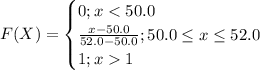
To determine the probability included between the values 51.9min and 50.9min, you have to calculate the difference between the accumulated probabilities until the right bond of the interval, 51.9min, and the accumulated probabilities until the left bond of the interval, 50.9min.
![P(50.9Using the expression for the cumulated probability between the maximum and minimum values of the distribution, you can calculate both accumulated probabilities:[tex]\begin{gathered} F(51.9)-F(50.9)=((51.9-50.0)/(52.0-50.0))-((50.9-50.0)/(52.0-50.0)) \\ ((1.9)/(2))-((0.9)/(2))=0.95-0.45=0.5 \end{gathered}]()
The probability that the length of a class chosen at random is between 50.9min and 51.9min is 0.5.