Formula to find the slope, m, or change in function of a graph is given below as,

For the first linear graph,
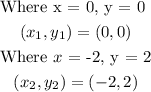
Substituting the coordinates into the equation,

For the second linear graph,

Substituting the coordinates into the equation,
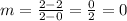
For the third linear graph,
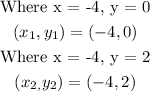
Substituting the coordinates into the equation,
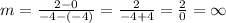
Hence, the slope of the first graph is -1.
Slope of the second graph is 0 since it doesn't rise vertically.
Slope of the third graph is infinite because it is a vertical line that neither move to the left or right.