Question

A bag contains 12 marbles. there are more red than green but taking green and blue together exceeds the number of reds. The total number of yellow and green marbles is more than the total number of red and blue. 1. Find exactly how many of each color there are in the bag. Explain your reasoning.2. How many different solutions can you find? Show how you know that you have found all solutions.

Answer 1

We have the following:

r = red marbles

g = green marbles

b = blue marbles

y = yellow marbles

the statement gives us the following information

`\begin{gathered} r+g+b+y=12 \\ r>g \\ g+b>r \\ y+g>r+b \end{gathered}`

now,

1.

We assume the lowest value for the green ones, that is, 1. For the rest we only have to assume values that conform to the conditions given in the statement

`\begin{gathered} g=1 \\ r=2 \\ b=2 \\ y=7 \end{gathered}`

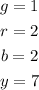
2.

There can only be 2 solutions and the other is the following:

`\begin{gathered} g=1 \\ r=2 \\ b=3 \\ y=6 \end{gathered}`

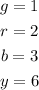
Those are all solutions since if we assume that g equals 2, the conditions cannot be met