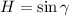Question

Prove Heron's formula

Answer 1

Given:

Heron's formula

Sol:

Heron's formula:

for finding the area of a triangle in terms of the lengths of its sides. In symbols, if a, b, and c are the lengths of the sides

In Heron's formula is:

`S=(a+b+c)/(2)`

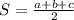
Heron's formula to find the area of a triangle is:

So, the area is:

`\text{ Area }=√(s(s-a)(s-b)(s-c))`

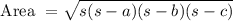
Area of triangle is :

`\begin{gathered} =(1)/(2)*\text{ base }*\text{ height} \\ \\ =(1)/(2)* b* h \end{gathered}`

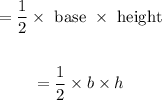
Use cosine law then:

`\begin{gathered} A=(1)/(2)ab\sin\gamma \\ \\ =(1)/(4)√(4a^2b^2-(a^2+b^2-c^2)^2) \\ \\ =(1)/(4)√((2ab-(a^2+b^2-c^2)(2ab+(a^2+b^2-c^2)) \\ \\ \end{gathered}`

Solve then:

`\begin{gathered} =(1)/(4)√((c^2-(a-b)^2)((a+b)^2-c^2)) \\ \\ =\sqrt{((c+(a-b))(c-(a-b))((a+b)-c)((a+b))+c)^(16))/(16)} \\ \\ =\sqrt{((b+c-a))/(2)((a+c-b))/(2)((a+b-c))/(2)((a+b+c)/(2)} \\ \\ =√(s(s-a)(s-b)(s-c)) \end{gathered}`

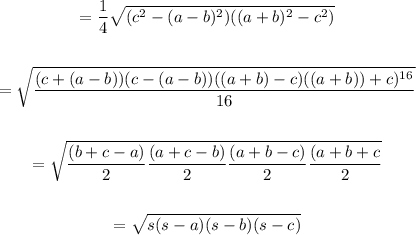
In cosine law.

Let us prove the result using the law of cosines:

Let a, b, c be the sides of the triangle and

`\alpha,\beta,\gamma`

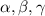
are opposite angle to the side.

We know that low of cosine is:

`\begin{gathered} \cos\gamma=(a^2+b^2-c^2)/(2ab) \\ \\ \text{ And} \\ \\ \sin\gamma=√(1-\cos^2\gamma) \\ \\ =(√(4a^2b^2-(a^2+b^2-c^2)))/(2ab) \end{gathered}`

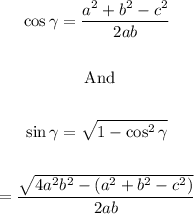
Here base of triangle = a

Height is:

`H=\sin\gamma`