Question

Can you help me with my work

Answer 1

Given expression for function :

`f(x)=x^2+6x+8`

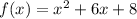
The general form of equation is :

`ax^2+bx+c=0`

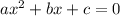
The axis of symmetry is define as : the line that divides the parabola into two equal halves,

i,e

`\text{ Ax is of symmetry = }(-b)/(2a)`

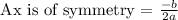
From the given expression we have a= 1, b =6, c=8

So,

Axis of symmtery = -6/2(1)

Axis of Symmetry = -3

i.e x = -3

Now for the vertex

Vertex : which is the minimum point of the parabola and the parabola opens upward.

for vertex

Substitute the x = -3 and solve for the valur of f(x)

`\begin{gathered} f(x)=x^2+6x+8 \\ f(-3)=(-3)^2+6(-3)+8 \\ f(-3)=9-18+8 \\ f(-3)=-9+8 \\ f(-3)=-1 \end{gathered}`

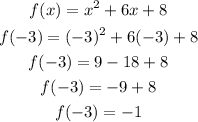
So the vertex will be (-3, -1)