Question

In the following diagram, A | B. 21 3x+7 A 22 23 B 24 26 25 4x+5 с 5y-29 27 28 3y+19 D 1. Use complete sentences to explain how the special angles created by the intersection of A and B by D can be used to solve for c. 2. Solve forc, showing all of your work. 3. Find the measure of Z6.

Answer 1

.

The intersection of the Lines A and B creates a pair of equivalent angles

This means

`\begin{gathered} \angle1=\angle4 \\ \angle3x+7=\angle6 \\ \angle2=\angle5 \\ \angle3=\angle4x+5 \end{gathered}`

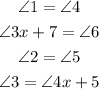
We can solve for x using the fact that the angles ∠3x + 7 and ∠3 form a linear pair. This gives us an equation and in it, we can substitute for ∠3 from the equations given above and solve the resulting equation for x.

(2).

We can solve for x using the fact that angles ∠3x + 7 and ∠3 form a linear pair. i.e .

`(3x+7)+\angle3=180^o`

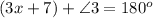
and since

`\angle3=\angle4x+5`

the above becomes

`(3x+7)+(4x+5)=180^o`

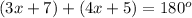
Expanding the above gives

`7x+12=180^o`

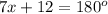
Subtracting 12 from both sides gives

`7x=168^o`

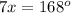
Finally, dividing both sides by 7 gives

`x=24.`

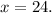
which is our answer!

(3).

Since we know that

`\angle3x+7=\angle6`

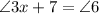
We can find the value of angle 6 by substituting the value of 3 in the above equation. This gives

`\begin{gathered} 3(24)+7=\angle6 \\ \end{gathered}`
`\boxed{\angle6=79^o\text{.}}`

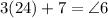
which is our answer!