Question

A chemical company makes 2 brands of antifreeze. the first brand is 35% pure antifreeze and the second is 85% pure aantifreeze. in order to obtain 70 gallons of a mixture that contains 80% pure antifreeze how many gallons of each brand must be used?

Answer 1

Let A be the amount of the brand of 35% pure antifreeze, and B the amount of the brand of 85% pure antifreeze.

Since a total of 70 gallons is to be obtained, then:

`A+B=70`

The total amount of pure antifreeze at the end should be 80% of 70, which is:

`(80)/(100)\cdot70=56`

On the other hand, the total amount of antifreeze in A gallons of the first brand and B gallons of the second brand is:

`(35)/(100)A+(85)/(100)B`

Therefore:

`0.35A+0.85B=56`

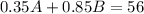
Isolate A from the first equation and substitute in the second one to find B:

`A=70-B`
`\begin{gathered} \Rightarrow0.35(70-B)+0.85B=56 \\ \Rightarrow24.5-0.35B+0.85B=56 \\ \Rightarrow0.5B=56-24.5=31.5 \\ \Rightarrow B=(31.5)/(0.5) \\ \Rightarrow B=63 \end{gathered}`

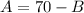
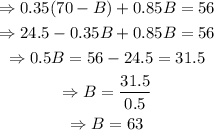
Substitute B=63 into the expression for A:

`\begin{gathered} A=70-63 \\ =7 \end{gathered}`

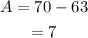
Therefore, to make a mixture of 70 gallons of 80% pure antifreeze, we need 7 gallons of the 35% pure brand and 63 gallons of the 85% pure brand.