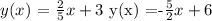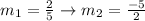Question

Are these lines parallel perpendicular or neither -2x + 5y = 15 5x + 2y = 12

Answer 1

If we have the equation of the from

`y=mx+b`

Then a line perpendicular to that equation is

`y=-(1)/(m)x+c`

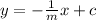
Let us bring each of the lines in slope-intercept form.

`\begin{gathered} -2x+5y=15 \\ 5y=15+2x \\ y=(2)/(5)x+3 \end{gathered}`

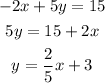
The other equation in slope-intercept form is

`\begin{gathered} 5x+2y=12 \\ 2y=12-5x \\ y=-(5)/(2)x+6 \end{gathered}`

As we can see the second equation is negative of the reciprocal of the second equation; therefore, the two lines are perpendicular.

`y(x)\text{ = }\frac{\text{2}}{5}x+3\text{ y(x) =-}(5)/(2)x+6`
`m_1=(2)/(5)_{}\rightarrow m_2=(-5)/(2)`