Question

The sum of the measures of any two supplementary angles is 180 degree. < L and < S are supplementary angles. The measures of < L is sixty-six degrees less than five times the measure of < S.What is the measure of < L? Use “deg” for the degree symbol.What is the measure of < S? Use “deg” for the degree symbol.

Answer 1

`\begin{gathered} m\angle L=139^0 \\ m\angle S=41^0 \end{gathered}`

Explanations:

If the sum of the measures of any two supplementary angles is 180 degrees and m
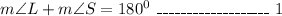`m\angle L+m\angle S=180^0\text{ \_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_\_ 1}`Also, if the measure of < L is sixty-six degrees less than five times the measure of < S, then:

`m\angle L=5m\angle S-66_(--------------)2`

Substitute equation 2 into equation 1 to have:

`(5m\angle S-66)+m\angle S=180`

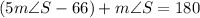
Simplify the result to have:

`\begin{gathered} 5m\angle S-66+m\angle S=180 \\ 5m\angle S+m\angle S-66=180 \\ 6m\angle S-66=180 \end{gathered}`

Add 66 to both sides of the resulting equation:

`\begin{gathered} 6m\angle S-66+66=180+66 \\ 6m\angle S=246 \end{gathered}`

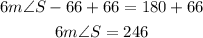
Divide both sides of the equation by 6

`\begin{gathered} \frac{\cancel{6}m\angle S}{\cancel{6}}=\frac{\cancel{246}^(41)}{\cancel{6}} \\ m\angle S=41^0 \end{gathered}`

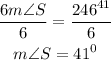
Get the measure of < L. Recall that:

`\begin{gathered} m\angle L=5m\angle S-66 \\ m\angle L=5(41)-66 \\ m\angle L=205-66 \\ m\angle L=139^0 \end{gathered}`

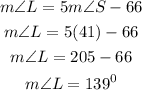
Hence the measure of < L is 139 deg. while the measure of