Question

. If a pair of parallel lines are rotated, which is true?

A) The lines remain parallel only if rotated 180°.
B) The lines remain parallel only if rotated 360°.
C) Rotated parallel lines always remain parallel lines.
D) Rotating parallel lines result in perpendicular lines.

Answer 1

Answer: The correct option is (C) Rotated parallel lines always remain parallel lines.

Step-by-step explanation: We are given ton select the correct statement about the rotation of two parallel lines.

We know that two parallel lines have same slope.

So, let m = 2 be the slope of the two parallel lines and 4, 7 are the y-intercepts.

Then, the equations of the lines are given by

`y=2x+4~~~~~~~~~~~~~~~~~~~~(i)\\y=2x+7~~~~~~~~~~~~~~~~~~~~(ii)`

Let us rotate the lines through an angle of 90° clockwise.

The co-ordinates of the point (x, y), after rotating 90° clockwise becomes (y, -x).

We have

P(-1, 2) and Q(1, 6) are two points on the line (i) and R(-1, 5) and S(1, 9) are two points on the line (ii).

So, after rotation, they become

P(-1, 2) ⇒ P'(2, 1),

Q(1, 6) ⇒ Q'(6, -1),

R(-1, 5) ⇒ R'(5, 1),

S(1, 9) ⇒ S'(9, -1).

So, slope of line (i) is

`m_1=(-1-1)/(6-2)=-(1)/(2),`

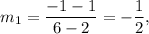
and the slope of line (ii) is

`m_2=(-1-1)/(9-5)=-(1)/(2).`

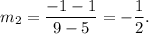
Since,
`m_1=m_2,`

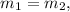
so the slopes of the lines after rotation is again same, and hence they are again parallel to each other.

So, the angle of rotation (90° or 180° or 360°)does not matter, the parallel lines will always be parallel.

Thus, the rotated parallel lines always remain parallel lines.

Option (C) is correct.

Answer 2

C.
Rotating parallel lines by the same amount does not change their parallel nature.